3240. Minimum Number of Flips to Make Binary Grid Palindromic II

Problem

You are given an m x n binary matrix grid.

A row or column is considered palindromic if its values read the same forward and backward.

You can flip any number of cells in grid from 0 to 1, or from 1 to 0.

Return the minimum number of cells that need to be flipped to make all rows and columns palindromic, and the total number of 1’s in grid divisible by 4.

https://leetcode.cn/problems/minimum-number-of-flips-to-make-binary-grid-palindromic-ii/

Example 1:

Input: grid = [[1,0,0],[0,1,0],[0,0,1]]
Output: 3
Explanation:

Example 2:

Input: grid = [[0,1],[0,1],[0,0]]
Output: 2
Explanation:

Example 3:

Input: grid = [[1],[1]]
Output: 2
Explanation:

Constraints:

m == grid.length
n == grid[i].length
1 <= m * n <= 2 * 10^5
0 <= grid[i][j] <= 1

Test Cases

1 2	class Solution: def minFlips(self, grid: List[List[int]]) -> int:

solution_test.py

import pytest

from solution import Solution


@pytest.mark.parametrize('grid, expected', [
    ([[1,0,0],[0,1,0],[0,0,1]], 3),
    ([[0,1],[0,1],[0,0]], 2),
    ([[1],[1]], 2),

    ([[1],[1],[1],[0]], 1),
])
class Test:
    def test_solution(self, grid, expected):
        sol = Solution()
        assert sol.minFlips(grid) == expected

Thoughts

3239. Minimum Number of Flips to Make Binary Grid Palindromic I 的进阶版。增加的要求除了行和列要同时是回文，还要求 1 的个数能被 4 整除。

翻转后的 grid，相对于中央水平线和垂直线是轴对称的。

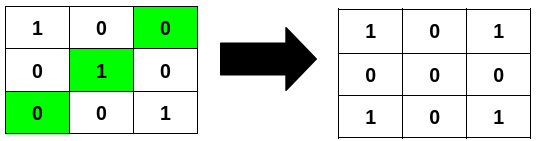
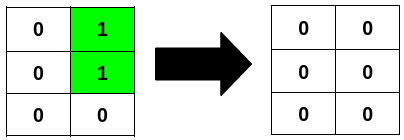
对于任意的 0 <= i < m // 2 和 0 <= j < n // 2，都需要 grid[i][j]、grid[i][-j-1]、grid[-i-1][j] 和 grid[-i-1][-j-1] 相等。不同 i、j 对应不同四格组相互独立，所以只要让每个四格组的翻转次数最少即可。如果四个格子的数字两两相同，则需要翻转两次；有一个和另外三个不同，需要翻转一次；四格全相同则不用翻转。（可以对四个格子的值求和，和为 2 则翻转两次，为 1 或 3 则翻转一次，为 0 或 4 则不翻转）。

显然只要四个格子的值相等，他们中 1 的个数要么是 0，要么是 4，都满足能被 4 整除的要求。

需要考虑 4 的整除性的是对于奇数行或奇数列的 grid，正中的那行或列，即下标为 m // 2（m 是奇数）的行或下标为 n // 2（n 是奇数）的列。

首先如果行和列同时是奇数，那么中央位置只有一个格子，其值必需为 0，如果原来是 1，需要翻转一次。

遍历正中行和列每一组对称的双格组，记录两个格子都是 1 的组数，如果是偶数，则 1 的个数能被 4 整除，否则就会余 2。再看一个 0 一个 1 的双格组，如果有，就把第一组里的 0 翻转成 1，跟前边的余 2 加起来正好是 4。剩下的一个 0 一个 1 的双格组，都把 1 翻转成 0。如果没有一个 0 一个 1 的双格组，需要把其边多的两个 1 都翻转成 0。

Code

solution.py

from typing import List


class Solution:
    def minFlips(self, grid: List[List[int]]) -> int:
        m = len(grid)
        n = len(grid[0])
        m_half = m >> 1
        n_half = n >> 1
        flip_count = 0

        # Four-cells groups.
        for i in range(m_half):
            for j in range(n_half):
                s = grid[i][j] + grid[i][-j-1] + grid[-i-1][j] + grid[-i-1][-j-1]
                flip_count += min(s, 4 - s)

        # Center cell.
        if m & 1 & n:
            flip_count += grid[m_half][n_half]

        # Two-cells pairs (in middle row and middle column).
        one_one = 0 # The number of 1-1 pairs (mod 2).
        zero_one = 0 # The number of 0-1 and 1-0 pairs.
        if m & 1:
            for j in range(n_half):
                s = grid[m_half][j] + grid[m_half][-j-1]
                one_one ^= (s >> 1)
                zero_one += (s & 1)

        if n & 1:
            for i in range(m_half):
                s = grid[i][n_half] + grid[-i-1][n_half]
                one_one ^= (s >> 1)
                zero_one += (s & 1)

        flip_count += zero_one if zero_one > 0 else (one_one << 1)
        return flip_count

本文除了题目描述（Problem）部分之外，其他内容由 Calf 原创，采用 CC BY-NC-SA 4.0 许可协议，转载请注明出处。